APPENDIX
Return times and resilience
It is important to distinguish between behavior near a stable
equilibrium and behavior near the boundary of a domain of attraction,
which is an unstable equilibrium or separatrix. As discussed in
Section 2, the long return times associated with a loss of resilience
are caused by slow dynamics near the unstable
equilibrium, not by slow dynamics near the stable
equilibrium point. Unfortunately, there are two conflicting
definitions of resilience and consequent confusion about the
connection between resilience and return times.
Pimm (1991:13) defines resilience as "how fast a variable that has
been displaced from equilibrium returns to it. Resilience could be
estimated by a return time, the amount of time taken for the
displacement to decay to some specified fraction of its initial
value.'' Pimm (1991: 33) describes return to equilibrium by the
equation

where  is the
population density at time
is the
population density at time 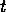 ,
, 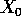 is the initial population density,
and
is the initial population density,
and  is the
equilibrium density. The differential equation for
is the
equilibrium density. The differential equation for  that corresponds to this formula is
that corresponds to this formula is

A similar model with discrete time could be given instead, but that
would not alter the following argument. If we measure displacement
from  by
by  , then
, then  satisfies
satisfies

which is equivalent to Eq. (A.1) if 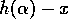 is replaced by
is replaced by  . Strictly speaking,
Pimm's definition depends upon this simplicity, because the amount of
time required for
. Strictly speaking,
Pimm's definition depends upon this simplicity, because the amount of
time required for  to decay to some specified fraction of its initial value is only
constant if the model (A.1) is used. In
fact, if the initial displacement is
to decay to some specified fraction of its initial value is only
constant if the model (A.1) is used. In
fact, if the initial displacement is 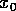 and the fraction is
and the fraction is 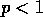 , then (A.1) implies that
, then (A.1) implies that

from which we conclude that the return time 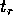 is given by
is given by

The remarkable feature is that the magnitude 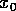 does not appear in this
formula. This is a feature of this model only, as we shall see
below. In more general circumstances, such a result can be expected to
hold only in the limit as
does not appear in this
formula. This is a feature of this model only, as we shall see
below. In more general circumstances, such a result can be expected to
hold only in the limit as 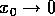 . Such results are called "local."
As pointed out in Section 2, a common error is to extrapolate local
results to global ones. In the present context, it amounts to
replacing a complicated function by a linear approximation. Such
approximations are certainly easy to work with, but they may miss
essential features of the dynamics. In fact, failure to recognize the
distinction between local stability and global stability can lead to
unwarrented optimism about the likely consequences of interventions in
natural systems. If we think that stability to small perturbations
necessarily implies stability to large perturbations, then precautions
are never required.
. Such results are called "local."
As pointed out in Section 2, a common error is to extrapolate local
results to global ones. In the present context, it amounts to
replacing a complicated function by a linear approximation. Such
approximations are certainly easy to work with, but they may miss
essential features of the dynamics. In fact, failure to recognize the
distinction between local stability and global stability can lead to
unwarrented optimism about the likely consequences of interventions in
natural systems. If we think that stability to small perturbations
necessarily implies stability to large perturbations, then precautions
are never required.
In order to distinguish behavior near the equilibrium at  from behavior near an
unstable equilibrium, we must use a model with more parameters than (A.2). We set
from behavior near an
unstable equilibrium, we must use a model with more parameters than (A.2). We set
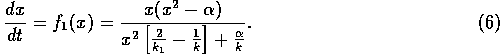
This particular form leads to an especially simple equation for the
return time: the time to reach a position 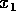 starting at
starting at 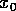 is given by
is given by

and the form for 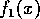 was chosen so that
was chosen so that
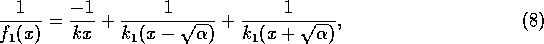
as can be verified algebraically. In view of (A.7) and (A.8),
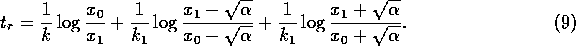
Now, if we replace 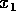 by
by 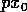 , (A.9)
becomes
, (A.9)
becomes
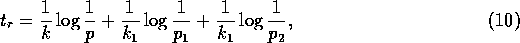
where

If the last two terms in (A.10) are
omitted, this result is identical to Pimm's assumption (A.1). Our more complicated dynamical
assumption (A.6) is the analogue of Pimm's
assumption if there are three equilibria. Under what conditions does
(A.10) imply large return times? The first
term, which corresponds to Pimm's model, implies a long return time if
the ratio 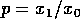 is
small or if
is
small or if 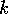 is
small. In Pimm's discussion,
is
small. In Pimm's discussion,  is a parameter that describes a
probe or observation of the system. Ordinarily,
is a parameter that describes a
probe or observation of the system. Ordinarily,  is fixed, and the return time
provides an estimate for
is fixed, and the return time
provides an estimate for 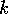 .
.
The second term in (A.10) implies a long
return time if 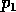 is
small or
is
small or  is
small. Our previous discussion was concerned with a possibly variable
is
small. Our previous discussion was concerned with a possibly variable
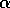 and disturbances
that might take the system near an unstable equilibrium. That
corresponds to
and disturbances
that might take the system near an unstable equilibrium. That
corresponds to 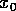 near
near  , or
, or 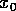 near
near 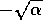 . In such a case,
. In such a case, 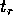 will be large even if the parameter
will be large even if the parameter
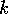 is large. That
is, return times may be long, even for systems that show very rapid
return when close to the stable equilibrium. According to this point
of view, long return times may be diagnostic for a small
is large. That
is, return times may be long, even for systems that show very rapid
return when close to the stable equilibrium. According to this point
of view, long return times may be diagnostic for a small 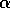 or for disturbances
that are large enough to take the system near an unstable
equilibrium. They may also correspond to weak repulsion from the
unstable equilibrium, i. e., small
or for disturbances
that are large enough to take the system near an unstable
equilibrium. They may also correspond to weak repulsion from the
unstable equilibrium, i. e., small  . If a disturbance takes the
system beyond the unstable equilibrium, there is no return at all.
. If a disturbance takes the
system beyond the unstable equilibrium, there is no return at all.
In summary, according to Pimm (1991) and according to us, long return
times may be diagnostic for a loss of resilience, but the meanings of
the terms are quite different in the two cases. Pimm is concerned with
behavior near a stable equilibrium. In that case, a long return time
for a given displacement from the equilibrium indicates a small
coefficient 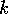 or,
equivalently, a small derivative of
or,
equivalently, a small derivative of 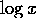 . We are concerned with behavior
of a system with two or three equilibria, one of which is
stable. Resilience describes the tendency of the system to return to
its stable equilibrium. A long return time is due to disturbances that
bring the system near an unstable equilibrium, or possibly to a weak
repulsion from an unstable equilibrium.
. We are concerned with behavior
of a system with two or three equilibria, one of which is
stable. Resilience describes the tendency of the system to return to
its stable equilibrium. A long return time is due to disturbances that
bring the system near an unstable equilibrium, or possibly to a weak
repulsion from an unstable equilibrium.

 is the
population density at time
is the
population density at time 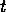 ,
, 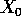 is the initial population density,
and
is the initial population density,
and  is the
equilibrium density. The differential equation for
is the
equilibrium density. The differential equation for  that corresponds to this formula is
that corresponds to this formula is

 by
by  , then
, then  satisfies
satisfies

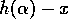 is replaced by
is replaced by  . Strictly speaking,
Pimm's definition depends upon this simplicity, because the amount of
time required for
. Strictly speaking,
Pimm's definition depends upon this simplicity, because the amount of
time required for  to decay to some specified fraction of its initial value is only
constant if the model (A.1) is used. In
fact, if the initial displacement is
to decay to some specified fraction of its initial value is only
constant if the model (A.1) is used. In
fact, if the initial displacement is 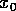 and the fraction is
and the fraction is 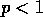 , then (A.1) implies that
, then (A.1) implies that

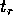 is given by
is given by

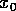 does not appear in this
formula. This is a feature of this model only, as we shall see
below. In more general circumstances, such a result can be expected to
hold only in the limit as
does not appear in this
formula. This is a feature of this model only, as we shall see
below. In more general circumstances, such a result can be expected to
hold only in the limit as 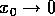 . Such results are called "local."
As pointed out in Section 2, a common error is to extrapolate local
results to global ones. In the present context, it amounts to
replacing a complicated function by a linear approximation. Such
approximations are certainly easy to work with, but they may miss
essential features of the dynamics. In fact, failure to recognize the
distinction between local stability and global stability can lead to
unwarrented optimism about the likely consequences of interventions in
natural systems. If we think that stability to small perturbations
necessarily implies stability to large perturbations, then precautions
are never required.
. Such results are called "local."
As pointed out in Section 2, a common error is to extrapolate local
results to global ones. In the present context, it amounts to
replacing a complicated function by a linear approximation. Such
approximations are certainly easy to work with, but they may miss
essential features of the dynamics. In fact, failure to recognize the
distinction between local stability and global stability can lead to
unwarrented optimism about the likely consequences of interventions in
natural systems. If we think that stability to small perturbations
necessarily implies stability to large perturbations, then precautions
are never required.
 from behavior near an
unstable equilibrium, we must use a model with more parameters than
from behavior near an
unstable equilibrium, we must use a model with more parameters than 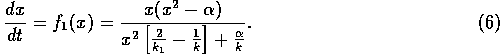
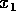 starting at
starting at 
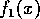 was chosen so that
was chosen so that
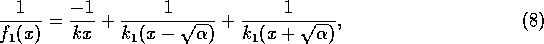
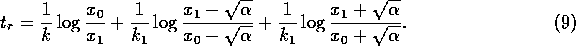
 ,
, 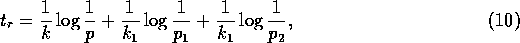

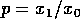 is
small or if
is
small or if 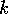 is
small. In Pimm's discussion,
is
small. In Pimm's discussion,  is a parameter that describes a
probe or observation of the system. Ordinarily,
is a parameter that describes a
probe or observation of the system. Ordinarily, 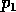 is
small or
is
small or  is
small. Our previous discussion was concerned with a possibly variable
is
small. Our previous discussion was concerned with a possibly variable
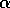 and disturbances
that might take the system near an unstable equilibrium. That
corresponds to
and disturbances
that might take the system near an unstable equilibrium. That
corresponds to  , or
, or 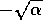 . In such a case,
. In such a case, 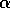 or for disturbances
that are large enough to take the system near an unstable
equilibrium. They may also correspond to weak repulsion from the
unstable equilibrium, i. e., small
or for disturbances
that are large enough to take the system near an unstable
equilibrium. They may also correspond to weak repulsion from the
unstable equilibrium, i. e., small 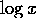 . We are concerned with behavior
of a system with two or three equilibria, one of which is
stable. Resilience describes the tendency of the system to return to
its stable equilibrium. A long return time is due to disturbances that
bring the system near an unstable equilibrium, or possibly to a weak
repulsion from an unstable equilibrium.
. We are concerned with behavior
of a system with two or three equilibria, one of which is
stable. Resilience describes the tendency of the system to return to
its stable equilibrium. A long return time is due to disturbances that
bring the system near an unstable equilibrium, or possibly to a weak
repulsion from an unstable equilibrium.